Solution:
Given that the height is expressed as
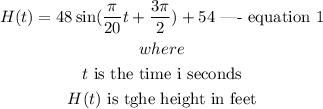
To find the maximum height,
step 1: Take the first derivative.
Thus,
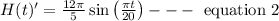
step 2: Find the critical point.
At the critical point, H(t)' equals zero.
Thus,
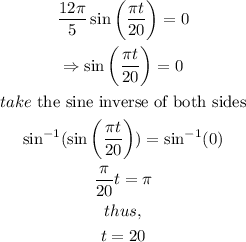
step 3: Take the second derivative.
Thus, we have
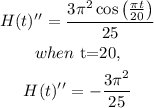
Since H(t)'' is negative, we have a maximum point.
To evaluate the maximum height, we substitute the value of 20 for t into the H(t) function.
Thus, we have
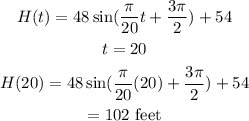
Hence, the correct option is