Answer:
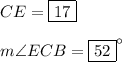
Explanation:
The diagonals of a rectangle bisect each other and are also of equal length
This means in the given rectangle ABCD with AC and BD as diagonals,
the following hold true:
AE = CE = 2x + 1
AC = 2(AE) = 2(2x + 1) = 4x + 2
BD = 3x + 10
Therefore since the diagonals are equal,
AC = BD
→ 4x + 2 = 3x + 10
→ 4x - 3x = 10 - 2
→ x = 8
CE = 2x + 1 = 2(8) + 1 = 17
BD = 3x + 10 = 3(8) + 10 = 24 + 10 = 34
BE = 1/2 BD= 1/2 (34) = 17
ΔBEC is an isosceles triangle with BE = CE and therefore ∠EBC = ∠ECB
The angles of a rectangle are 90°
We are given m∠EBA = 28°
So m∠EBC = 90 - 28 = 52°
m∠ECB = m∠EBC
m∠ECB is also 52°