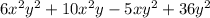Question

Simplify. Write the expression such that the terms are in descending order with respect to the power of x and the variables within each term are in alphabetical order. 5xy(2x−y)+6y2(x2+6)

Answer 1

Answer:
`6x^2y^2 + 10x^2y-5xy^2+36y^2`

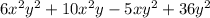
Step-by-step explanation:

First, you would distribute each term by multiplying 5xy with 2x, 5xy with -y, and so on. Assuming the "y2" in "6y2" is supposed to be y^2, this is what it would look like:

`10x^2y - 5xy^2+6x^2y^2+36y^2`

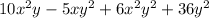
Then, you would have to arrange it with respect to the power of x (start with the largest exponent of x) with variables in alphabetical order. This means the variables should be xy rather than yx.

`6x^2y^2 + 10x^2y-5xy^2+36y^2`