Answer: Here is the answers to your previous question that was deleted. As for this question, I believe the answer is the first one.
1)
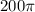
2)
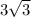
3)

4) 9
5) 0.151
Explanation:
#1
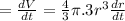
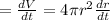
![=(dV)/(dt) =4\pi r^2*2...[(dr)/(dt) =2]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ysk7tqbxi1hyqe87jimnh20iw1m8w4ncxw.png)
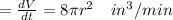
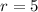
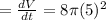
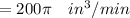
#2
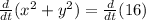
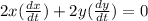
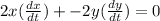
Divide both sides by 2.
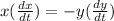
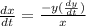
As the particle is passing through the point
 , So, the point will satisfy the equation. Substituting the value of
, So, the point will satisfy the equation. Substituting the value of
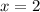 ,
,
 and
and
 ,
,
we get ,

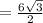
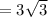
#3
Go on to Desmos and put in
 and look at x=0 and x=1 and use those two as your points.
and look at x=0 and x=1 and use those two as your points.
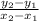
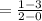
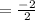

#4
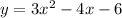
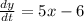
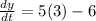
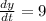
#5
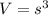
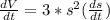

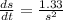
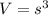

 ≈
≈

Substitute equation2 to equation1, we get
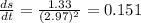
I hope this helps you! Cheers! ^^