Answer:
The proportion of faculty that earns more than $25,250 but less than $38,750 is 0.8025.
Explanation:
Chebyshev Theorem
The Chebyshev Theorem can also be applied to non-normal distribution. It states that:
At least 75% of the measures are within 2 standard deviations of the mean.
At least 89% of the measures are within 3 standard deviations of the mean.
An in general terms, the percentage of measures within k standard deviations of the mean is given by
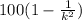 .
.
In this question, we have that:
Mean of 32000, Standard deviation of 3000.
Using Chebyshev's Theorem, what is the proportion of faculty that earns more than $25,250 but less than $38,750
This is within 38,750 - 32,000 = 32,000 - 25,250 = $6,750 of the mean.
Value of k:
This is k standard deviations from the mean. k is given by:
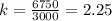
Proportion:
The percentage is:
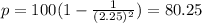 .
.
So the proportion is 0.8025