Question

A wallet contains 33 notes, all of which are either $5 or $10 notes. If it amounts to $240, how many $10 notes are there?

Answer 1

Number of $5 notes = 18

Number of $10 notes = 15

Explanation:

Framing and solving system of equations:

Let the number of $5 notes be 'x' and the number of $10 notes be 'y'.

Total number of notes = 33

x + y = 33 -------------------(I)

The value of $5 notes = 5*x = 5x

The value of $10 notes = 10 *y = 10y

Total amount = $240

5x + 10y = 240

Divide the entire equation by 5,

`\sf (5)/(5)x+(10)/(5)y=(240)/(5)\\\\`

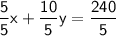
x + 2y = 48 -----------------------(II)

Multiply the equation (I) by (-1) and then add to equation(I) and so the x will be eliminated, and we can find the value of 'y'.

(I)* (-1) -x - y = -33

(II) x + 2y = 48 {Now add}

y = 15

`\boxed{\bf y = 15}`

Substitute y = 15 in equation(I)

x + 15 = 33

x = 33 - 15

x = 18

`\boxed{\bf x = 18}`

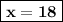
Number of $5 notes = 18

Number of $10 notes = 15