Question

I need a step by step walkthrough on finding the y-coordinate of the vertex of a quadratic equation. This is the equation:5x^2 +8x -13Things I already know:a= 5b= 8c= -13x= -4/5The axis of symmetry is -4/5.I need a very clear, easy to understand answer.

Answer 1

First, let's remember what the axis of symmetry means.

The axis of symmetry is a line that splits our parabola in half. This line also has a very interesting property: The vertex belongs to this line.

(illustrative example. Not the given parabola)

Now, for the particular parabola we're given, we know that this axis of symmetry is:

`x=-(4)/(5)`

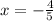
Notice that, taking into account what we already know about the defnition of the axis of symmetry, we can conclude that this is the x-coordinate of the vertex.

To find the corresponding y-value, we just plug it in the formula of our parabola:

`\begin{gathered} y=5x^2+8x-13 \\ \\ \rightarrow y=5\cdot(-(4)/(5))^2+8\cdot(-(4)/(5))-13 \\ \\ \rightarrow y=5\cdot(-(4)/(5))^{}\cdot(-(4)/(5))+8\cdot(-(4)/(5))-13 \\ \\ \rightarrow y=5\cdot((16)/(25))^{}+8\cdot(-(4)/(5))-13 \\ \\ \rightarrow y=(16)/(5)^{}-(32)/(5)-13 \\ \\ \rightarrow y=(16)/(5)^{}-(32)/(5)-(65)/(5) \\ \\ \rightarrow y=(16-32-65)/(5)^{} \\ \\ \Rightarrow y=-(81)/(5) \end{gathered}`

Therefore, we can conclude that the y-coordinate of our vertex is:

`y=-(81)/(5)`

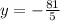
(Given parabola, with its axis of symmetry and vertex highlighted)