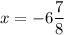Question

Please I need help Asp please help me solve the equation in the picture below also please show steps on how to solve

Answer 1

`x=-6(7)/(8)`

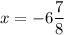
Explanation:

`Solve\:9\:(1)/(4)\:+\:x\:=\:2\:(3)/(8)`

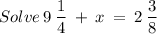
Step 1:
Convert all mixed fractions to improper fractions.
This will make it easier to add and subtract

Mixed fraction
`a(b)/(c)` can be converted to an improper fraction using

`a(b)/(c)=(a\cdot \:c+b)/(c)`

`9(1)/(4)=(9\cdot 4+1)/(4) = (37)/(4)`

`2(3)/(8)=(2\cdot 8+3)/(8) =(19)/(8)`

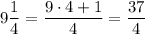
Step 2:
Replace every mixed fraction in the original equation with its equivalent improper fraction:

`(37)/(4)+x=(19)/(8)`

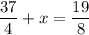
Step 3
Solve for x

• 
  `\mathrm{Move}\:(37)/(4)\:\mathrm{to\:the\:right\:side}`:
  
  `(37)/(4)+x-(37)/(4)=(19)/(8)-(37)/(4)`
  
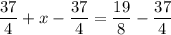
• Simplify:
  
  `x =(19)/(8)-(37)/(4)`
  To calculate the right side value, multiply
  `(37)/(4)` by 2 on both numerator and denominator. This will get the denominator as 8 for both fractions and we can simply subtract the numerator:
  
  
  `\begin{aligned}x & = (19)/(8) - (37 * 2 )/(4 * 2) \\ & = (19)/(8) - (74)/(8)\\& = (19 - 74)/(8)\\& = - (55)/(8)\\\end{aligned}\\\\`
  
• 
  `\mathrm{Convert\:to\:mixed\:number:\:Quotient(Remainder)/(Divisor)}`
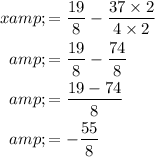
  55 goes into 8 six times so the whole part(quotient) is 6
  Remainder = 55 - 8 x 6 = 55 - 48 = 7
  So the fractional part = remainder/divisor = 7/8
  
• Result:
  
  `x=-6(7)/(8)`