Question

10. In this university, among all students, 15% are senior, 25% are junior, 25% are sophomore, and so 35% are freshmen. Among senior, 40% have scholarship; among junior, 30% have scholarship; among sophomore, 20% have scholarship, and among freshmen, 10% have scholarship. Among those have scholoarship, what is the percentage of studens who are senior

Answer 1

27.27% of the students with scolarship are seniors.

Explanation:

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this question:

Event A: Has scolarship

Event B: Is a senior

15% are senior, and of those, 40% have scolarship. So

`P(A \cap B) = 0.15*0.4 = 0.06`

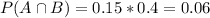
Probability of a scolarship:

15% of 40%(seniors)

30% of 25%(juniors)

20% of 25%(sophmores).

10% of 35%(freshmen). So

`P(A) = 0.15*0.4 + 0.3*0.25 + 0.2*0.25 + 0.1*0.35 = 0.22`

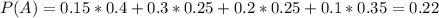
Percentage:

`P(B|A) = (P(A \cap B))/(P(A)) = (0.06)/(0.22) = 0.2727`

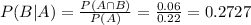
0.2727*100 = 27.27%

27.27% of the students with scolarship are seniors.