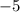Question

Doing indices rules and need help!

Answer 1

n =
`-5`

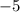
Explanation:

Two Laws of indices will be applied here:

`(a^(m))/(b^(m)) = a^(m - n)`

`a^(m)` ×
`a^(n)` =
`a^(m + n)`

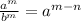
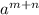
Applying the above stated laws on the left side of the equation and the resulting simplified term will then be equated to the right side of the equation:

`(y^(4)* y^(n))/(y^(2))` =
`(y^(4 + n))/(y^(2))`

=
`y^((4 + n) - 2)` =
`y^(-3)`

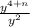
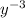
It can be observed that the bases in both sides of the equation are the same, therefore their respective indices (or powers) are equal to each other:

`(4 + n) - 2 = -3`

Expand the parenthesis and bring all the like terms together:

`4 + n - 2 = -3`

`n = -3 + 2 - 4`

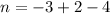
n is isolated and made the subject of the equation. Thus, we are able to solve for n:

n =
`-5`