Answer:
F = 233.52 N, θ' = 351.41º
Step-by-step explanation:
In this exercise we must find the net force applied on the donkey.
For this we use Newton's second law, where we create a reference frame with the horizontal x axis
let's decompose the forces
Jack
= 80.5 N
Jill
cos 45 = F_{2x} / F₂2
sin 45 = F_{2y} / F₂2
F_{2x} = F₂ cos 45
F_{2y} = F₂ sin 45
F_{2x} = 81.7 cos 45 = 57.77 N
F_{2y} = 81.7 sin 45 = 57.77 N
Jane
cos (270 + 45) = F_{3x} / F₃3
sin 315 = F_{3y} / F₃
F_{3x} = 131 cos 315 = 92.63 N
F_{3y} = 131 sin 315 = -92.63 N
the force can be found in each axis
X axis
F_{x} = F_{1x} + F_{2x} + F_{3x}
F_{x} = 80.5 +57.77 + 92.63
F_{x} = 230.9 N
Axis y
F_{y} = F_{1y} + F_{2y} + F_{3y}
F_{y} = 0 + 57.77 -92.63
F_{y} = -34.86 N
we can give the result in two ways
a) F = (230.9 i ^ - 34.86 j ^) N
b) in the form of module and angle
we use the Pythagorean theorem
F = √(Fₓ² + F_{y}²
F = √(230.9² + 34.86²)
F = 233.52 N
let's use trigonometry for the angle
tan θ =
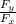
θ = tan⁻¹ (\frac{F_y}{F_x} })
θ = tan⁻¹ (-34.86 / 230.9)
θ = -8.59º
if we measure this angle from the positive side of the x-axis counterclockwise
θ' = 360 -θ
θ‘= 360- 8.59
θ' = 351.41º