Question

URGENT HELP WILL GIVE 50 POINTS IF ANSWERED RIGHT !! given right triangle abc with altitude bd drawn to hypotenuse ac if ad=4 and bd=2 what is the length of dc​

Answer 1

The length of DC is 1 unit.

Explanation:

To calculate the length of DC, use the Geometric Mean Theorem (Altitude Rule).

Geometric Mean Theorem - Altitude Rule

The altitude drawn from the vertex of the right angle perpendicular to the hypotenuse separates the hypotenuse into two segments. The ratio of the altitude to one segment is equal to the ratio of the other segment to the altitude.

`\boxed{\sf (Altitude)/(Segment\:1)=(Segment\:2)/(Altitude)}`

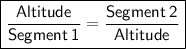
From inspection of the given right triangle:

• Altitude = BD = 2
• Segment 1 = AD = 4
• Segment 2 = DC = x

Substitute the values into the formula and solve for x:

`\implies (2)/(4)=(x)/(2)`

`\implies 2 \cdot 2 = 4 \cdot x`

`\implies 4=4x`

`\implies x=1`

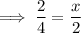
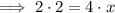
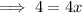
Therefore, the length of DC is 1 unit.