Answer:
Stop the line of production since the sample mean (56.96) is outside the confidence interval [
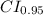 = ( 56.98, 57.05 )]
= ( 56.98, 57.05 )]
Explanation:
Given that;
95% confidence interval for these parts is 56.98 to 57.05
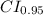 = ( 56.98, 57.05 )
= ( 56.98, 57.05 )
Sample mean = 56.96
We know that;
If the sample mean is within the confidence interval then, our decision is to keep the line operating as it is inside the confidence interval.
But if the sample mean is not within the confidence interval them, our decision is to stop the line of production as it is outside the confidence interval.
Now since our mean sample (56.96) does not lie between with the 95% confidence interval
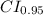 = ( 56.98, 57.05 ).
= ( 56.98, 57.05 ).
Therefore, Stop the line of production since the sample mean (56.96) is outside the confidence interval [
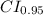 = ( 56.98, 57.05 )]
= ( 56.98, 57.05 )]