The ladder and the house wall form a right triangle given by the scheme below
Where x represents the distance between the base of the ladder and the house wall.
After moving the ladder 6 ft farther, a new triangle is generated with the following measures
where h represents how far up the ladder is on the house wall.
Using the Pythagorean theorem on both triangles, which states that “In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides“, we get the following system.
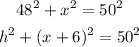
Solving the first equation for x, we have
![\begin{gathered} 48^2+x^2=50^2 \\ x^2=50^2-48^2 \\ x^2=(50+48)(50-48) \\ x^2=98\cdot2 \\ x^2=196 \\ x=\sqrt[]{196} \\ x=14 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/n389nh5nrjvqa4jh62vr.png)
Using this x value on the second equation, we can determinate the height of the second triangle.
![\begin{gathered} h^2+(14+6)^2=50^2 \\ h^2+20^2=50^2 \\ h^2=50^2-20^2 \\ h^2=(50+20)(50-20) \\ h^2=70\cdot30 \\ h=\sqrt[]{2100} \\ h=10\sqrt[]{21} \\ h=45.8257569496\ldots \\ h\approx45.8 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/p31ifuc647epsk9467p4.png)
After moving the base 6ft farther, the ladder will reach 45.8ft far up the side of the house.