Question

What is the value of x

Answer 1

x = 12

Explanation:

Pythagoras Theorem

= Hypotenuse² = adjacent² + opposite²

From the question

Hypotenuse = 20

Adjacent = x

Opposite = 16.

The value for x

= 20² = x² + 16²

= 400 = x² + 256

= 400-256 = x²

= 144 = x²

`√(144) = { √(x) }^(2) \\ √(144) = x \\ 12 = x`

Therefore adjacent (X) = 12

Answer 2

The value of x is 12.

Greetings!!!

Firstly, recall the Pythagoras' theorem states that for all right-angled triangles, 'The square on the hypotenuse is equal to the sum of the squares on the other two sides'. The hypotenuse is the longest side and it's always opposite the right angle. In this triangle a² = b²+ c² and angle is a right angle.

a, b, and c represent the lengths of sides of a triangle, then: a2+b2=c2 if and only if the triangle is a right triangle.

Thus, on this question

the hypotenuse is a= 20

the value of b= 16

so the required value is c

`a {}^(2) = b {}^(2) + c {}^(2)`

substitute known variables into the equation

`(20 ){}^(2) = (16) {}^(2) +( c) {}^(2)`

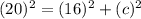
evaluate both sides

`400 = 256 +( c) {}^(2)`

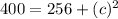
minus 256 from both sides

`400 - 256 = (c) {}^(2)`

`144 = (c) \frac{2}{}`

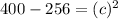
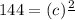
write bothe sides under radical in order to erase the square

`√(144) = \sqrt{c {}^(2) }`

solve for c

`c = 12`

Hope it helps