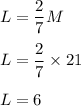Answer:
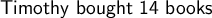
Explanation:
Let's use first letters of names to represent the number of books bought by that person. This makes it easier to explain.
Therefore, the books bought by each of the three persons are T(imothy), L(aurence) and M(ike)
We will now convert each of the word description into math equations and solve
We are given that the ratio of the number of books bought by Laurence and Mike were 2: 7
We can write this as:

Multiplying both sides by M:

Timothy and Laurence bought a total of 20 books can be represented as
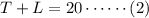
Timothy and Mike bought a total of 35 books can be represented as
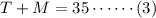
Eq (3) - Eq (2):
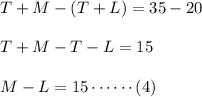
Substituting for
 from equation (1) into equation (4) we get
from equation (1) into equation (4) we get
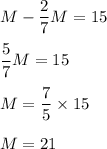
Given
 using equation (3),
using equation (3),
 we get
we get
 (Answer)
(Answer)
If we wanted to find out how many books Laurence bought use Eq(1)