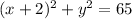Question

9. Write the equation of a circle that has a center at (-2, 0) and an endpoint at (-9,-4).Make sure your answer is 4-5 sentences.

Answer 1

The equation of a circle with radius r and center (h,k) is:

`(x-h)^2+(y-k)^2=r^2`

If the center is (-2,0) and (-9,-4) is an endpoint, use the distance between two points formula to find the distance from the center to and endpoint. That distance is the radius of the circle:

`\begin{gathered} r=\sqrt[]{(-9-(-2))^2+(-4-0)^2} \\ =\sqrt[]{(-9+2)^2+(-4)^2} \\ =\sqrt[]{(-7)^2+(-4)^2} \\ =\sqrt[]{49+16} \\ =\sqrt[]{65} \\ \Rightarrow r^2=65 \end{gathered}`

Substitute h=-2, k=0 and r^2=65 to find the equation of the circle:

`\begin{gathered} (x-(-2))^2+(y-(0))^2=65 \\ \Rightarrow(x+2)^2+y^2=65 \end{gathered}`

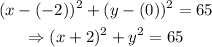
Therefore, the equation of the circle is:

`(x+2)^2+y^2=65`