The density property of real numbers says that there is always a real number between two other real numbers.
Between the number 1 and 2, some examples of this are 1.252,
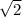 , 1.5,
, 1.5,
 , etc.
, etc.
An application of this is the intermediate value theorem, which states that in a continuous function on interval whose domain contains the interval
[a, b], then any given value between f(a) and f(b) exists at some point within the interval.
We can say that if I am on a swing, and a function gives my height, there is an infinite amount of real numbers between my minimum height and maximum height. If I swing 7 feet off the ground, and return to 1 foot height, at some point I was 4 feet in the air,
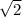 feet in the air, or
feet in the air, or
 feet in the air.
feet in the air.
Hope this helps,
Jeron
:- )