Answer:
10 m
Explanation:
We are given that the relationship between intensity of sound and distance from the source is given by
Intensity (I)=
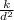
Where I=Intensity
d=Distance of sound from the source
If distance d=1m
Intensity, I=0.1

We have to find how close she can to the stage if vanessa does not want the sound intensity to be more than 0.001(w)/(m^(2)).
Using the formula


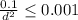
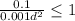

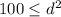


Hence, she can sit 10 m away from the stage.