Question

A slow 24-hour clock loses 25 minutes a day. At noon on the first of October, it is set to show the correct time. When will this clock next show the correct time?

Answer 1

Nsjdjs. Shshshsbbd dnsiebshdine didieo

Answer 2

Nov 27 at 14:24 hours

Explanation:

• First let's figure out exactly when the slow clock will synchronize with a correct clock

• At the rate of loss of 25 minutes per day, let x be the number of days when the two clocks coincide

• The total time lost in x days = 25x minutes

• The clocks will synchronize when the total time lost is exactly 24 hours.
  24 hours = 24 x 60 minutes = 1440 minutes

• Setting this equal to 25x we get
  25x = 1440
  
• 
  `x = \rm{{\frac{1440 \textrm{ minutes}}{25 \textrm{ min/day}} = 57.6 \;days}`
  

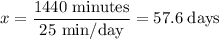
57.6 days equates to 57 days, 14 hours, 24 minutes (using a calculator)

Adding to Oct 1 at 12:00:00 we get this as Nov 27 at 14:24 hours