Question

List the elements of the universal set U, the set of natural numbers less than 15. (Enter your answer in roster notation.)

A = {3, 5, 6, 7, 9, 12}

B = {1, 2, 7, 11}

U = {1,2,3,4,5,6,7,8,9,10,11,12,13,14}
Correct: Your answer is correct.

Help me with step 3 please


Step 3
The set we want, the complement of
A ∪ B,
is the set which contains all the elements which are in U, but not in
A ∪ B.
(Enter your answer in roster notation.)


(A ∪ B)' =

Answer 1

(A ∪ B)' = {4, 8, 10, 13, 14}

Explanation:

Set Notation

`\begin{array}c \cline{1-3} \sf Symbol & \sf N\:\!ame & \sf Meaning \\\cline{1-3} \{ \: \} & \sf Set & \sf A\:collection\:of\:elements\\\cline{1-3} \cup & \sf Union & \sf A \cup B=elements\:in\:A\:or\:B\:(or\:both)}\\\cline{1-3} \cap & \sf Intersection & \sf A \cap B=elements\: in \:both\: A \:and \:B} \\\cline{1-3} \sf ' & \sf Complement & \sf A'=elements\: not\: in\: A \\\cline{1-3} \sf \text{U} & \sf Universal& \textsf{Set of all possible values}\\\cline{1-3} \end{array}`

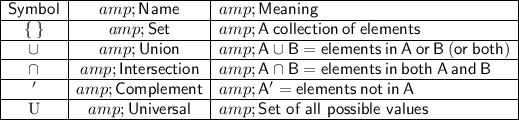
Given sets:

• A = {3, 5, 6, 7, 9, 12}
• B = {1, 2, 7, 11}

If the universal set, U, is the set of natural numbers less than 15 then:

• U (universal) = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14}

(A ∪ B) means the union of sets A and B.

Therefore, (A ∪ B) is the combination of the elements of the two sets A and B.

(A ∪ B)' means the complement of (A ∪ B).

Therefore, (A ∪ B)' is everything that is not in the union of sets A and B.

`\begin{aligned}\sf (A \cup B)^'&=\text{U}-\sf (A \cup B)\\&=\{1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14\}-(\{3, 5, 6, 7, 9, 12\} \cup \{1, 2, 7, 11\})\\&=\{1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14\}-\{1, 2, 3, 5, 6, 7, 9, 11, 12\}\\&=\{4, 8, 10, 13, 14\}\end{aligned}`