Question

There are 3 counters in a bag. One counter is red. One counter is green. One counter is blue. Mike takes at random a counter from the bag. He puts the counter back in the bag. Then Ellie takes at random a counter from the bag. The possible combinations are: RR, RG, RB, GR, GG, GB, BR, BG, BB. Find the probability that Mike takes a blue counter and then Ellie takes a green counter.

Answer 1

`(1)/(9)` or 11.11%

Explanation:

P(A) = probability of an event

f = favored outcomes

N = number of total outcomes

`P(A)=(f)/(N)`

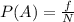
In this equation there is one favored income, when the first counter is blue and the second one is green. There are also nine total outcomes. This means that the equation necessary is:

`P(A)=(1)/(9)`

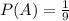
The probability that Mike takes a blue counter and then Ellie takes a green counter is
`(1)/(9)` or around 11.11%