Question

Use the formula V=s^3 to find the volume of a cube with the sides of length s= 2 1/4 inches

Answer 1

6.375 cubic inches

Explanation:

To find the volume of a cube using the formula V = s^3, where s represents the length of one side, we simply need to raise s to the power of 3.

Given that the length of one side, s, is 2 1/4 inches, we need to first convert the fractional part to decimal form:

`\sf:\implies{2 1/4 inches = 2 + 1/4 inches}`

`\sf:\implies{= 2.25 inches}`

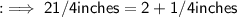
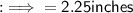
Now, we can use this value in the formula:

`\sf:\implies{V = s^3 = 2.25^3}`

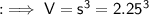
To raise a number to the power of 3, we simply multiply the number by itself three times:

V = 2.25 × 2.25 × 2.25 = 6.375 cubic inches

Therefore, the volume of the cube with sides of length 2 1/4 inches is 6.375 cubic inches.