Question

What is an equation of the line that passes through the point (−1, −6) and is

perpendicular to the line x + 6y=
6?

Answer 1

y-6x=0 or y = 6x

Explanation:

To find the slope of the second line, we can use the formula:

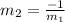`m_(2)=(-1)/(m_(1) )`, where m2 is the slope of the second line (unknown) and m1 is the slope of the first line (given/known)

Currently the line x + 6y = 6 is in standard form and we can convert it to slope-intercept form (y = mx + b) to find the slope:

`x+6y=6\\6y=6-x\\y=(6-x)/(6)\\ y=1-1/6x`

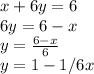
Thus, the slope of first line (m1) is -1/6.

Now we can use this value to find the slope of the second line (m2):

`m_(2)=-1/(-1/6)\\ m_(2)=6`

Since we found the slope, now we must find the y-intercept of the line by plugging in the slope and the point we're given:

`-6=6(-1)+b\\-6=-6+b\\0=b`

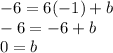
Thus, the equation of the line in slope intercept form is y = 6x

To convert it to standard form like the first line, we simply subtract 6x to get

y-6x = 0