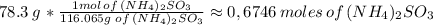Question

How many moles are in 78.3g of ammonium sulfite?

Answer 1

0,6746 moles of ammonium sulfite

Step-by-step explanation:

(NH₄)₂SO₃ - ammonium sulfite
`\equiv \, N_2H_8SO_3`

Atomic weight of each element:

N = 14.00 * 2 = 28

H = 1.00 * 8 = 8

S = 32.065 * 1 = 32.065

O = 16 * 3 = 48

Total atomic weight = 28 + 8 + 32.065 + 48

Total atomic weight = 116,065 gr/mol

`78.3\,g \,* (1mol\,of\, (NH_4)_2SO_3)/(116.065g \, \,of \, (NH_4)_2SO_3 ) \approx 0,6746\, moles \, of \, (NH_4)_2SO_3`

Answer 2

To find the number of moles in 78.3 grams of ammonium sulfite, you would divide the mass by the molar mass of the substance. The molar mass of ammonium sulfite is (14.0067 + 14.0067 + 32.065 + 64.0638) g/mol = 124.1472 g/mol.

78.3 g / 124.1472 g/mol = 0.63 moles

Step-by-step explanation: