Question

Describe each x-intercept as "crosses" or "touches" the x-axis

Answer 1

Third option

• touches at x = 1
• crosses at x = -3
• crosses at x = -5

Explanation:

If the polynomial has a factor of the form
`(x - h)^p,` the behavior of its graph is determined by the value of p. p .

One zero of the polynomial is x = h

x - h is a zero of multiplicity p

The following is a summary of rules regarding multiplicities and x-intercepts.

• For even multiplicities, i.e. p = 2, 4, 6, 8,... the graph will touch the x-axis for the zeros
• For odd multiplicities, i.e. p = 1, 3, 5, 7,... the graph will cross the x-axis for the zeros

The given polynomial is:

`\mbox {\large \textsf(x - 1)^2(x+3)(x + 5)^5}}`

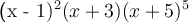
The zeros can be found by setting each of these factors to 0 and solving for x
x - 1 = 0 ==> x = 1
x + 3 = 0 ==> x == -3

x + 5 = 0 ==> x = -5

Hence, the zeros of this polynomial are

• x = 1 with a multiplicity of 2
• x = -3 with a multiplicity of -3
• x = -5 with a multiplicity of -5

Using the rules provided we see that the graph

• touches at x = 1
• crosses at x = -3
• crosses at x = -5
  

This corresponds to
Third option

• 
  
•