Question

Two cyclists leave towns 255 kilometers apart at the same time and travel toward each other. One cyclist travels 7 kmh slower than the other. If they meet in 5 hours, what is the rate of each cyclist?

Answer 1

Let x be the speed of the faster cyclist and y be the speed of the slower cyclist.
We know that:
x = y + 7 (because one cyclist travels 7 km/h slower than the other)

Also,
distance = rate x time
the distance between the two towns is 255km, and the time is 5 hours.
so
255 = (x+y) * 5/2

now we can substitute the first equation into the second one:
255 = (y+7 +y) * 5/2

Now we have a system of two equations with two variables:
x = y + 7
255 = (2y + 7) * 5/2

Solving for y in the first equation:
y = x - 7

substitute this equation into the second equation:
255 = (2(x-7) + 7) * 5/2

solving for x:
x = (2552)/(52+7) = 24 km/h

then y = x - 7 = 24 - 7 = 17 km/h

So the faster cyclist travels at 24 km/h and the slower cyclist travels at 17 km/h.

Answer 2

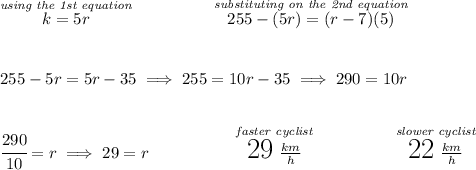
Check the picture below.

r = rate of the faster cyclist

r - 7 = rate of the slower cyclist

we know they both met 5 hours later, so the time each one has been cycling has been 5 hours for each.

let's say the faster cyclist on those 5 hours covered "k" kilometers, that means the slower one cover the slack, namely "255 - k" kilometers.

`{\Large \begin{array}{llll} \underset{distance}{d}=\underset{rate}{r} \stackrel{time}{t} \end{array}} \\\\[-0.35em] ~\dotfill\\\\ \begin{array}{lcccl} &\stackrel{Km s}{distance}&\stackrel{km/h}{rate}&\stackrel{hour}{time}\\ \cline{2-4}&\\ \textit{faster cyclist}&k&r&5\\ \textit{slower cyclist}&255-k&r-7&5 \end{array}\hspace{5em} \begin{cases} k=(r)(5)\\\\ 255-k=(r-7)(5) \end{cases} \\\\[-0.35em] ~\dotfill`

`\stackrel{\textit{using the 1st equation}}{k=5r}\hspace{5em}\stackrel{\textit{substituting on the 2nd equation}}{255-(5r) = (r-7)(5)} \\\\\\ 255-5r=5r-35\implies 255=10r-35\implies 290=10r \\\\\\ \cfrac{290}{10}=r\implies 29=r\hspace{5em}\stackrel{\textit{faster cyclist}}{\text{\LARGE 29}~(km)/(h)}\hspace{5em}\stackrel{\textit{slower cyclist}}{\text{\LARGE 22}~(km)/(h)}`