Question

a test has 20 questions and is worth 100 points. the test consists of x true/false questions worth 4 points and y multiple choice questions worth 8 points each. How many of each type of question are on the test?

Answer 1

Translate the word problem into a system of equations, with x representing true/false questions and y representing multiple choice questions:

(1)
`x+y=20` <-- a test has 20 total questions

(2)
`4x+8y=100` <-- the test is worth 100 total points

Now we have a system of two equations with two unknowns, which we can solve with the elimination method.

Let's multiply equation (1) by -4 so that we can eliminate x from the system:

`-4x-4y=-80`

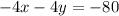
Now, add this new equation to equation (2) to eliminate x so that we only have one variable left:

`4y=20`

Solve for y:

`y=5`

Now, substitute this value for y into either the original equation (1) or (2). I will do equation 1 for simplicity:

`x+5=20`

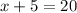
Solve for x:

`x=15\\`

Notice that we now have values for both x and y, so we are done. We now know that there are 15 true/false questions and 5 multiple choice questions on the test!