Question

In the diagram, the radius of the outer circle is 2x em and the radius of the inside circle is 6 cm. The area of the shaded region is 220 cm? What is the value of x2​

Answer 1

Answer: 8 (second choice)

Explanation:

Area of a Circle

Given a circle of radius r, the area is calculated by the formula:

`A=\pi\ r^2`

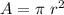
There are two circles in the diagram. The outer circle has a radius of r1=2x, thus its area is:

`A_1=\pi\ (2x)^2`

The interior circle has a radius of r2=6 cm, thus its area is:

`A_2=\pi\ 6^2=36\pi`

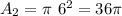
The shaded area is obtained by subtracting A1-A2:

`A=\pi\ (2x)^2-36\pi`

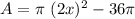
The value of the shaded area is given as 220π cm2. Equating:

`\pi\ (2x)^2-36\pi=220\pi`

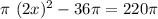
Dividing by π:

`(2x)^2-36=220`

Adding 36:

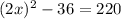
`(2x)^2=220+36=256`

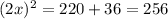
Taking square root:

`2x=√(256)=16`

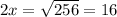
Dividing by 2:

x = 8 cm

Answer: 8 (second choice)