Question

85 POINTS, NEED HELP ASAP

Answer 1

To find:-

• The arc length.

Answer:-

We are here given that, an arc subtends an angle of 11π/6 radians on the centre of a circle whose radius is 18cm . We that the if r is the radius of a circle and
`\theta` is the angle substended by an arc at the centre then, the length of the arc is given by,

`\implies \green{ \ell =(\theta)/(2\pi )* 2\pi r}\\`

where ,

• 
  `\theta` is the angle in radians .
• 
  `\ell` is the arc length.

Now on substituting the respective values, we have;

`\implies \ell =((11\pi)/(6))/(2\pi )* 2\pi * 18\ cm\\`

`\implies \ell =(11\pi)/(6)* 18\ cm\\`

`\implies \ell = 33\pi \\`

`\implies \ell = 33* 3.14\\`

`\implies \underline{\underline{\green{\ell = 103.62\ cm }}}\\`

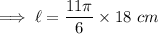
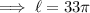
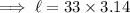
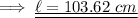
Hence the length of the arc is 103.62 cm .

and we are done!

Answer 2

arc length ≈ 104 cm

Explanation:

arc length is calculated as

arc = circumference of circle × fraction of circle

= 2πr ×
`((11\pi )/(6) )/(2\pi )` ( r is the radius )

cancel 2π on denominator of fraction with the 2π multiplier

arc = r ×
`(11\pi )/(6)`

= 18 ×
`(11\pi )/(6)`

= 3 × 11 × π

≈ 104 cm ( to the nearest whole number )