Question

How do I find the perpendicular line of y = -3x + 2. The perpendicular line passes through the point 6, 8 (please break down into steps)

Answer 1

y =
`(1)/(3)`x + 6

Explanation:

The equation we currently have right now is y = -3x+2. Since we know that the line equation is y = mx + b, let's take out m to find the slope of a perpendicular line. M will be -3.

To convert a regular slope to a perpendicular one, we have to:

- reciprocal/flip it (this means that if m = 3, the perpendicular will be
`(1)/(3)`)

- swap the signs (this will mean that if a number is positive, then it will become negative)

Therefore, if m = -3, the perpendicular slope will be
`(1)/(3)` (we flipped and changed signs)

Now, we are also given that this line passes through the point (6,8), so we can use this formula:
`y - y1 = m(x-x1)`

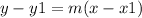
What do we know right now?

- y1 = 8 (from (6,8))

- x1 = 6 (from (6,8))

- m =
`(1)/(3)`

Let's plug in what we know:

y - 8 =
`(1)/(3)`(x - 6)

y =
`(1)/(3)`(x-6) + 8

y =
`(1)/(3)`x - 2 + 8

y =
`(1)/(3)`x + 6