Given:
Consider the total age of 5 children with age difference 3 years is 50 years.
To find:
The age of the youngest and oldest child.
Solution:
Let the ages of the 5 children are x-6, x-3, x, x+3, x+6.
Sum of their ages is 50 years.

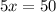

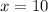
The age of the youngest child is
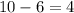
The age of the oldest child is
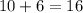
Therefore, the age of youngest child is 4 years and the age of the oldest child is 16 years.