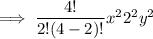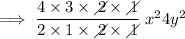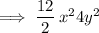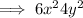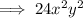Question

In two or more complete sentences, prove how to find the third term of the expansion of

(x+2y)^4

Answer 1

-2

Explanation:

y = −2− x 2 y = - 2 - x 2

Answer 2

`24x^(2)y^2`

Explanation:

`\boxed{\begin{minipage}{5cm} \underline{Binomial Theorem}\\\\$\displaystyle (a+b)^n=\sum^(n)_(k=0)\binom{n}{k} a^(n-k)b^(k)$\\\\\\where \displaystyle \binom{n}{k} = (n!)/(k!(n-k)!)\\\end{minipage}}`

We can use the Binomial Theorem to find any term of a binomial expansion.

The first term is when k = 0, so the third term is when k = 2.

Compare the given expression (x + 2y)⁴ with the formula to find the values of a, b and n.

Therefore:

• a = x
• b = 2y
• n = 4
• k = 2

Substitute the values into the formula to find the third term:

`\implies \displaystyle\binom{4}{2}x^(4-2)(2y)^2`

`\implies (4!)/(2!(4-2)!)x^(2)2^2y^2`

`\implies (4 * 3* \diagup\!\!\!\!2* \diagup\!\!\!\!1)/(2* 1* \diagup\!\!\!\!2* \diagup\!\!\!\!1)\;x^(2)4y^2`

`\implies (12)/(2)\:x^24y^2`

`\implies 6x^(2)4y^2`

`\implies 24x^(2)y^2`