Answer:

Explanation:
Given

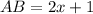
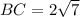
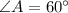
Required
Find x
To find x, we make use of cosine formula which states:
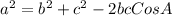
In this case:
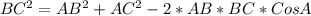
Substitute values
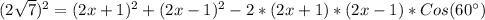
Evaluate all exponents
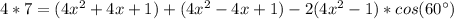
Open brackets
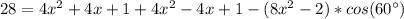
Collect Like Terms
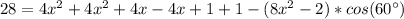

Substitute 0.5 for cos(60)
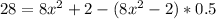
Open bracket
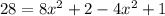
Collect Like Terms
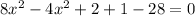
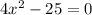
Collect Like Terms
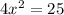
Divide both sides by 4
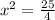
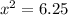
Take positive square root of both sides

