Question

Identify the quadratic form of
4(x-1)^2-9(x-1)=2. Than factor and solve for x.

Answer 1

see explanation

Explanation:

4(x - 1)² - 9(x - 1) =- 2

let t = x - 1 , then rewrite the expression as

4t² - 9t = - 2 ( add 2 to both sides )

4t² - 9t + 2 = 0 ← in standard form

Step 2

consider the factors of the product of the coefficient of the t² term and the constant term which sum to give the coefficient of the t- term

product = 4 × 2 = 8 and sum = - 9

the factors are - 8 and - 1

use these factors to split the t- term

4t² - 8t - t + 2 = 0 ( factor first/second and third/fourth terms )

4t(t - 2) - 1(t - 2) = 0 ← factor out (t - 2) from each term

(t - 2)(4t - 1) = 0 ← in factored form

equate each factor to zero and solve for t

4t - 1 = 0 ⇒ 4t = 1 ⇒ t =
`(1)/(4)`

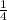
t - 2 = 0 ⇒ t = 2

Step 3

Now solve for x using

t = x - 1 ( add 1 to both sides ), then

x = t + 1

t =
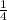`(1)/(4)` , then x =
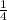`(1)/(4)` + 1 =
`(5)/(4)`

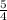
x = 2 , then x = 2 + 1 = 3