Question

Write this expression so that its denominator, if any, is a positive integer. \[\frac{2}{3 + \sqrt{7}}\].

Answer 1

3 -√7

Explanation:

You want to rationalize the denominator of 2/(3 +√7).

Rationalize the denominator

The denominator of such an expression is turned to an integer by multiplying numerator and denominator by the conjugate of the denominator.

`(2)/(3+√(7))=(2)/(3+√(7))\cdot(3-√(7))/(3-√(7))=(2(3-√(7)))/(3^2-7)=\boxed{3-√(7)}`

__

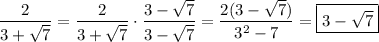
Additional comment

This process takes advantage of the factorization of the difference of squares:

a² -b² = (a +b)(a -b)

If 'b' is a value that can be made a rational real number by squaring it, then this multiplication by the conjugate can achieve that end. The conjugate of a sum is the corresponding difference.

This process is also used with complex numbers, since i² = -1, a real number.