Question

Please solve and show how!​

Answer 1

x = 0 and x = 16

Explanation:

You want the solution to √(2x+4) -√x = 2.

Solution

We eliminate the radicals by squaring until there aren't any. After the first squaring, we have to separate the radical term from the others.

`√(2x+4)-√(x)=2\\\\(2x+4)-2√((2x+4)(x))+(x)=4\qquad\text{square both sides}\\\\3x=2√(x(2x+4))\qquad\text{add $√(x(2x+4))-4$}\\\\9x^2=4(2x^2+4x)\qquad\text{square both sides}\\\\x^2-16x=0\qquad\text{subtract the right side from both sides}\\\\x(x -16) = 0\qquad\text{factor}`

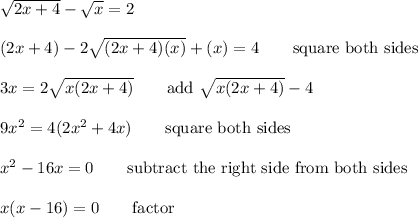
Zero product rule

The solutions to this are the values of x that make the factors zero. Those values are x=0 and x=16. Trying these in the original equation, we have ...

√(2·0 +4) -√0 = 2 -0 = 2 . . . . . . x=0 is a solution

√(2·16 +4) -√16 = 6 -4 = 2 . . . . . x=16 is a solution

The two solutions to the equation are x=0 and x=16.