Answer:
a) Possible values of m and n:
- m = 23, n = 0
- m = 21, n = 1
- m = 19, n = 2
- m = 17, n = 3
- m = 15, n = 4
- m = 13, n = 5
- m = 11, n = 6
- m = 9, n = 7
- m = 7, n = 8
- m = 5, n = 9
- m = 3, n = 10
- m = 1, n = 11
b) 9 two-legged animals and 7 four-legged animals.
c) Either 1 four-legged animal or 2 two-legged animals have been added to the exhibit.
Explanation:
Given expression:
2m + 4n = 46
where:
- m = 2 legged animals
- n = 4 legged animals
Part a
Calculate the greatest number of four-legged animals by dividing the total number of animals by 4:
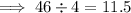
Therefore, the greatest number of four-legged animals is 11.
When there are no four-legged animals, n = 0.
Substitute n = 0 into the given equation:

Therefore, there are 23 two-legged animals when there are no four-legged animals.
Each time the number of four-legged animals increases by 1, the number of two-legged animals decreases by 2.
Therefore, the possible values for m and n so that the total number of legs is 46 are:
- m = 23, n = 0
- m = 21, n = 1
- m = 19, n = 2
- m = 17, n = 3
- m = 15, n = 4
- m = 13, n = 5
- m = 11, n = 6
- m = 9, n = 7
- m = 7, n = 8
- m = 5, n = 9
- m = 3, n = 10
- m = 1, n = 11
Part b
If the number of two-legged animals is 2 more than the number of four-legged animals then:
Therefore, there are 9 two-legged animals and 7 four-legged animals.
Part c
If the number of legs in the exhibit increases by 4, then either:
- 1 four-legged animal has been added to the exhibit, or
- 2 two-legged animals have been added to the exhibit.