Define the exponential function
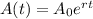
where A is the hourly wage.
Given that A(0) = 7.50 and A(10) = 12.22.
By using definition of A(t),
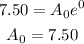
Then
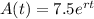
Now, use A(10)=12.22.
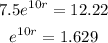
Take logarithm on both sides.
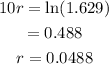
Substitute the value of r in A(t).
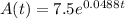
Find the wage after 10 more years.
Take t = 20 and substitute into A(t).
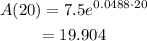
In 20 years, the daily wage will be $19.904.