Question

F(x) = √(x + 7), g(x) = √(x - 7)
Find the domain of f.

Answer 1

`x\ge -7`

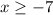
Explanation:

Square Root Function:

The square root function has a domain consisting of numbers greater than or equal to zero. This excludes negative numbers since the square root of a number, when multiplied by the same square root of a number results in the original number:
`y=√(x)\implies y*y=x`. If we wanted the square root of a negative number, we would need a number which multiplies by it self to get a negative number, but as you may recall, if we square a real number, we always get a positive. So negative numbers are not included in the domain (although there are imaginary solutions)

So we just need the inside of the square root to be greater than or equal to zero. Thus we can derive:
`x+7\ge 0` and then just subtract 7 from both sides to simplify:
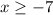`x\ge -7` and now we have our domain in interval notation.