Question

I just wnated to finish this im stressed.

Answer 1

`\boxed{\displaystyle f^(-1)(x) =\left((x-2)/(4)\right)^5 + 1\\}`

This is the last choice on the screenshot you provided

Explanation:

Use y to represent f(x)

`y=4\sqrt[5]{x-1}+2`

Replace x with y and y with x:

`x=4\sqrt[5]{y-1}+2`

Solve for y in terms of x and that will be the inverse of f(x)

• Switch sides:
  
  `4\sqrt[5]{y-1}+2=x`
  
• Subtract 2 from both sides:
  
  `4\sqrt[5]{y-1}=x-2`
  
  
• Divide both sides by 4
  
  `\sqrt[5]{y-1}=(x-2)/(4)`
  
• Raise both sides to the 5th power to get rid of the radical
  
  `\displaystyle \left(\sqrt[5]{y-1}\right)^5=\left((x-2)/(4)\right)^5`
  
  
• This works out to
  
  `\displaystyle y - 1 =\left((x-2)/(4)\right)^5\\`
  
  
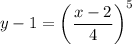
• Simplifying we get
  
  `\displaystyle y =\left((x-2)/(4)\right)^5 + 1\\`
  
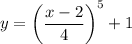
• The right side is the inverse of f(x)
  

Answer: Last choice on the screen, namely


`\boxed{\displaystyle f^(-1)(x) =\left((x-2)/(4)\right)^5 + 1\\}`