Question

How would you write `\sqrt{36}=6` as a logarithmic equation?Important Note: to type a logarithm, start with the word log and to add the base use the underscore symbol _For example, to get `\log_{5}x=y` I typed this into an equation:"log_5 x=y"Underscore is SHIFT+MINUS on most keyboards.

Answer 1

Step-by-step explanation:

Given;

We are given the following equation;

`√(36)=6`

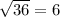
Required;

We are now required to write this as a Logarithmic equation.

Step-by-step solution;

To do this, we would first take the left side of the equation and simplify the radical;

`√(36)=36^{(1)/(2)}`

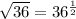
We now re-write the equation as shown below;

`36^{(1)/(2)}=6`

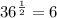
Now we can apply the log rule which is;

`\begin{gathered} If: \\ log_ba=c \\ Then: \\ b^c=a \end{gathered}`

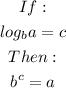
For example;

`\begin{gathered} If: \\ log_(10)100=2 \\ Then: \\ 10^2=100 \end{gathered}`

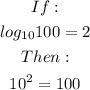
Therefore we would now have;

`\begin{gathered} For\text{ }the\text{ }equation: \\ 36^{(1)/(2)}=6 \end{gathered}`

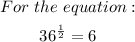
ANSWER:

`log_(36)6=(1)/(2)`