Question

Given parallelogram PQRS, with vertices P(-2,3), Q(3,8), R(4,1), and S(-1,-4) use slope to prove the parallelogram is a rhombus.

Answer 1

start by ubicating the points in the cartesian plane

To identify if the points given is a rhombus the slope for the opposite sides must be equal in order for the sides to be parallel

Also the diagonals of the figure must be perpendicular between them.

FIND SLOPES FOR PS AND QR

`m=(y_2-y_1)/(x_2-x_1)`

apply for both sides

`\begin{gathered} mPS=(-4-3)/(-1-(-2)) \\ mPS=(-7)/(1)=-7_{} \end{gathered}`
`\begin{gathered} mQR=(1-8)/(4-3) \\ mQR=-(7)/(1)=-7 \end{gathered}`

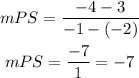
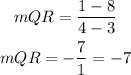
since both both sides have the same slope they are parallel.

FIND SLOPES FOR RS AND PQ

`\begin{gathered} mRS=(-4-1)/(-1-4) \\ mRS=(-5)/(-5)=1 \end{gathered}`
`\begin{gathered} mPQ=(8-3)/(3-(-2)) \\ mPQ=(5)/(5)=1 \end{gathered}`

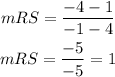
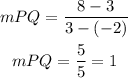
since both both sides have the same slope they are parallel.

FIND SLOPES FOR DIAGONAS QS AND PR

`\begin{gathered} mPR=\frac{3_{}-1_{}}{_{}-2-4} \\ mPR=(2)/(-6)=-(1)/(3) \end{gathered}`
`\begin{gathered} mQS=(8-(-4))/(3-(-1)) \\ mQS=(12)/(4)=3 \end{gathered}`

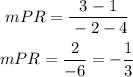
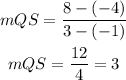
Find the product between the slopes

`(-1)/(3)\cdot3=-1`

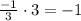
since the product of the slopes is -1, lines are perpendicular

Parallelogram PQRS is rhombus.