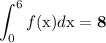Answer: 8
========================================================
Step-by-step explanation:
The left-most rectangle spans from x = 0 to x = 2. It has base 2 and height 2, which means it has an area of 2*2 = 4 square units. Let A = 4 since we'll use it later.
The middle rectangle goes from x = 2 to x = 4. It has base 2 and height 3 (because it goes from y = 0 to y = -3). The area is B = 2*3 = 6
Draw a vertical line through 6 on the x axis. This forms the final rectangle we need. It has base 2 (because it goes from x = 4 to x = 6) and height 5. The area is C = 2*5 = 10.
The small sliver to the right of x = 6 is ignored completely.
--------------------
Summary so far:
Those represent the areas of the rectangles from left to right. We ignore the portion to the right of x = 6.
Since rectangle B is below the x axis, we treat this as a negative area, or we subtract off this area. The positive areas of rectangles A and C are added.
So,
A-B+C = 4-6+10 = 8 is the final answer
We can write