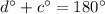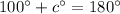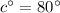GEOMETRY: I might just be slow but I don't understand what this question is asking at all. Can someone help? - QAmmunity.org

GEOMETRY: I might just be slow but I don't understand what this question is asking at all. Can someone help?

asked Nov 11, 2024 177k views

1 Answer

Answer:

b = 90

d = 100

c = 80

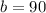
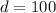
Explanation:

The problem is asking for the values of the variables
,
, and
in the given parallelogram with angles
$d\textdegree$ ,
$c\textdegree$ ,
$(b-10)\textdegree$ , and
$(b+10)\textdegree$ .

To solve for these variables, we can construct three equations using the knowledge that any two adjacent angles of a parallelogram are supplementary (their measures add to 180º).

The first equation shows that the top two angles are supplementary:

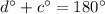
$d\textdegree + c\textdegree = 180\textdegree$

The second equation shows that the bottom two angles are supplementary:

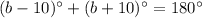
$(b-10)\textdegree + (b+10)\textdegree = 180\textdegree$

The third equations shows that the left two angles are supplementary:

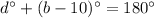
$d\textdegree + (b-10)\textdegree = 180\textdegree$

First, we can solve for
in the second equation using simple algebraic manipulation.

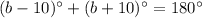
$(b-10)\textdegree + (b+10)\textdegree = 180\textdegree$

$(b+b)\textdegree + (10-10)\textdegree = 180\textdegree$

$2b\textdegree = 180\textdegree$

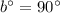
$b\textdegree = 90\textdegree$

b = 90

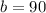
Using this
-value, we can solve for
by rewriting the third equation, plugging
in for
:

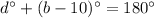
$d\textdegree + (b-10)\textdegree = 180\textdegree$

$d\textdegree + (90-10)\textdegree = 180\textdegree$

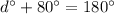
$d\textdegree + 80\textdegree = 180\textdegree$

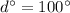
$d\textdegree = 100\textdegree$

d = 100

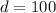
Finally, we can solve for
in the first equation by plugging
100 in for
:

$d\textdegree + c\textdegree = 180\textdegree$

$100\textdegree + c\textdegree = 180\textdegree$

$c\textdegree = 80\textdegree$

c = 80

Spajus answered Nov 15, 2024

by Spajus

8.0k points

Search QAmmunity.org