Answer:
√259 or 16.09
Explanation:
Hello!
I'll be labeling the vertices so that it is easier to understand the sides.
These are both right triangles, and we can find the measures of right triangles (given two other sides) by using the Pythagorean theorem:
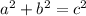
- a = leg
- b = leg
- c = hypotenuse (leg opposite to 90°)
In Triangle BCD, we have the measures of the hypotenuse and a leg. We can use that to find the missing side: CB
Since DB is the hypotenuse, it would be side c, and we can say that CD can be side a.
Solve for CB:
CB in simplest radical form is 5√21.
We can now solve for x, because now in triangle ABC, we have the measures of two sides: hypotenuse AC and leg CB.
Solve for AB:
AB in simplest radical form is √259, which is approximately 16.09.
The solution for x is √259 or 16.09.