Question

Can u help me with this

Answer 1

D) (6.6, 7.4)

Explanation:

Begin by finding the margin of error based on the given information.

`\boxed{\begin{minipage}{7.3cm}\underline{Margin of Error formula}\\\\$\textsf{Margin of Error}= Z * (S)/(√(n))$\\\\where:\\\phantom{ww} $\bullet$ $Z =$ $Z$ score\\\phantom{ww} $\bullet$ $S =$ Standard Deviation of a population\\\phantom{ww} $\bullet$ $n =$ Sample Size\\\end{minipage}}`

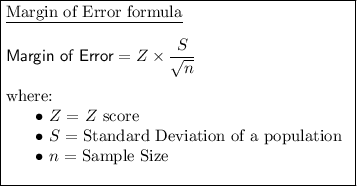
Given:

• S = 1.3
• n = 50

The z-score for 95% confidence level is 1.96.

Therefore, to find the margin of error, substitute the values into the formula:

`\implies \textsf{Margin of Error}= 1.96 * (1.3)/(√(50))`

`\implies \textsf{Margin of Error}=0.360341...`

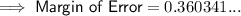
To find a reasonable range for the true mean number of hours a teenage spend on their phone, subtract and add the margin of error to the given mean of 7 hours:

`\begin{aligned}\implies \textsf{Lower bound}&=7-0.360341...\\&=6.63965...\\&=6.6\;\; \sf (1\;d.p.)\end{aligned}`

`\begin{aligned}\implies \textsf{Upper bound}&=7+0.360341...\\&=7.360341...\\&=7.4\;\; \sf (1\;d.p.)\end{aligned}`

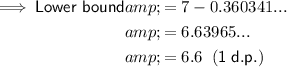
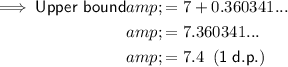
Therefore, the reasonable range for the true mean is:

• (6.6, 7.4)