Answer:
y = 2 ± √x is the inverse but it's not a function. (See note below for additional info)
Explanation:
Given the quadratic function:
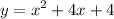
We can rewrite the function above using perfect square form:
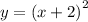
Finding an inverse, we swap the terms of x and y:
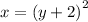
Square root both sides, solving for y:

Subtract both sides by 2:
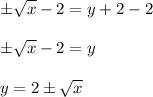
Therefore the inverse of y = x² + 4x + 4 is y = 2 ± √x.
Note: While it's possible to find the inverse of quadratic function, but the inverse version itself is not really a function. So if you want to go by definition or theorem, you can say there's no inverse function. Otherwise, the answer above is the solution.