Question

m(x) =2-x and n(x) =1+x^2Given:minimum x and Maximum x: -9.4 and 9.4minimum y and maximum y: -6.2 and 6.2Use m(x)/n(x)a) what are the zeroes?b) are there any asymptotes? c) what is the domain and range for this function?d) it it a continuous function?e) are there any values of y= m(x)/n(x) that are undefined? Explain

Answer 1

Given m(x) and n(x), the rational function m(x)/n(x) is

`f(x)=(m(x))/(n(x))=(2-x)/(1+x^2)`

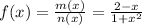
a) The zeroes of the function are the values of x such that f(x)=0; then,

`\begin{gathered} f(x)=0 \\ \Rightarrow(2-x)/(1+x^2)=0 \\ \Rightarrow2-x=0 \\ \Rightarrow x=2 \end{gathered}`

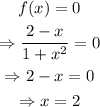
The zero of the function is at x=2.

b) Notice that the limits when x->+/- infinite are

`\begin{gathered} \lim _(x\to-\infty)f(x)=\lim _(x\to-\infty)-(x)/(x^2)=-\lim _(x\to-\infty)\frac{1}{x^{}}=-((1)/(-\infty))=(1)/(\infty)=0 \\ \text{and} \\ \lim _(x\to\infty)f(x)=\lim _(x\to\infty)-(x)/(x^2)=-\lim _(x\to\infty)\frac{1}{x^{}}=-((1)/(\infty))=-(1)/(\infty)=0 \end{gathered}`

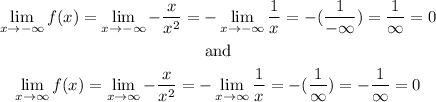
This implies that there is an asymptote at y=0; however, notice that

`\begin{gathered} f(x)=-0.1 \\ \Rightarrow x=3,7 \end{gathered}`

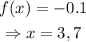
Thus, the graph of f(x) crosses y=0; thus, there are no asymptotes even if the behavior of the function tends to zero.

c) The function's domain consists of every value of x such that the denominator of f(x) is different than zero. Notice that 1+x^2>=1 since x^2>=0; thus

`\text{domain(f(x))}=\mleft\lbrace x\in\R\mright\rbrace=(-\infty,\infty)`

d) n(x) and m(x) are polynomial function; thus both functions are continuous. Then,

`\begin{gathered} f(x)=(m(x))/(n(x))\to\text{ is continuous for every x such that }n(x)\\e0 \\ \text{and} \\ n(x)\\e0,\text{ always} \\ \Rightarrow f(x)\to\text{continuous for every x} \end{gathered}`

Therefore, the rational function is indeed continuous for any value of x.

e) As we stated before, f(x) is defined for every value of x such that n(x) is different than zero, and n(x)>1 for every x. Thus, there are no values of x such that m(x)/n(x) is undefined.

f) As for the end behavior of the function, we found that the limits when x approaches +/- infinite are equal to zero. Therefore, the end behavior of the function is not positive nor negative since 0=-0