Question

Consider the quadratic function 1(x) = #x2 - 5x + 12. Which statements are true about the function and its

graph? Select three options.

A. The value of f(-10) = 82

B. The graph of the function is a parabola.

C. The graph of the function opens down.

D. The graph contains the point (20, -8).

E. The graph contains the point (0, 0).

Answer 1

B. The graph of the function is a parabola.

C. The graph of the function opens down.

E. The graph contains the point (0, 0).

Explanation:

The reason why option (A) is incorrect is because f(-10)
`\\eq` 82. We can see:
`= x^2 - 5x + 12\\= (-10)^2 - 5(-10) + 12\\= 100 + 50 + 12\\= 162 \\eq 82`

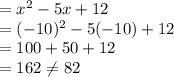
The reason why option (D) is incorrect because the graph does not contain the point (20, -8). You can verify this by plugging the x-value of 20 into the equation for f(x) and solving for y: